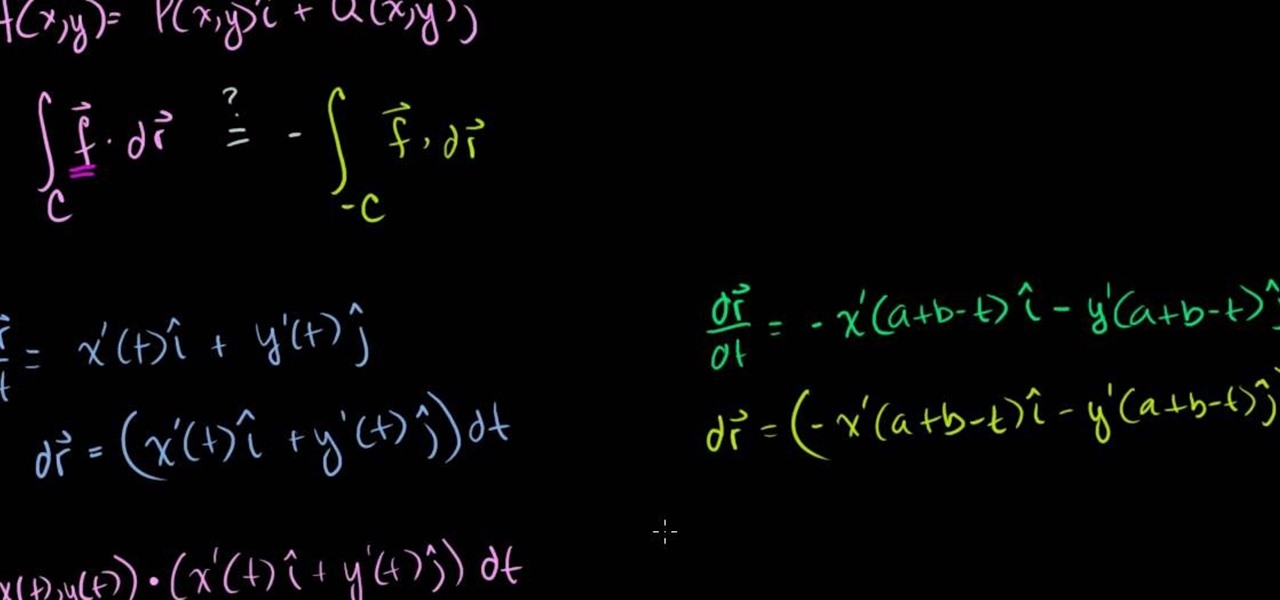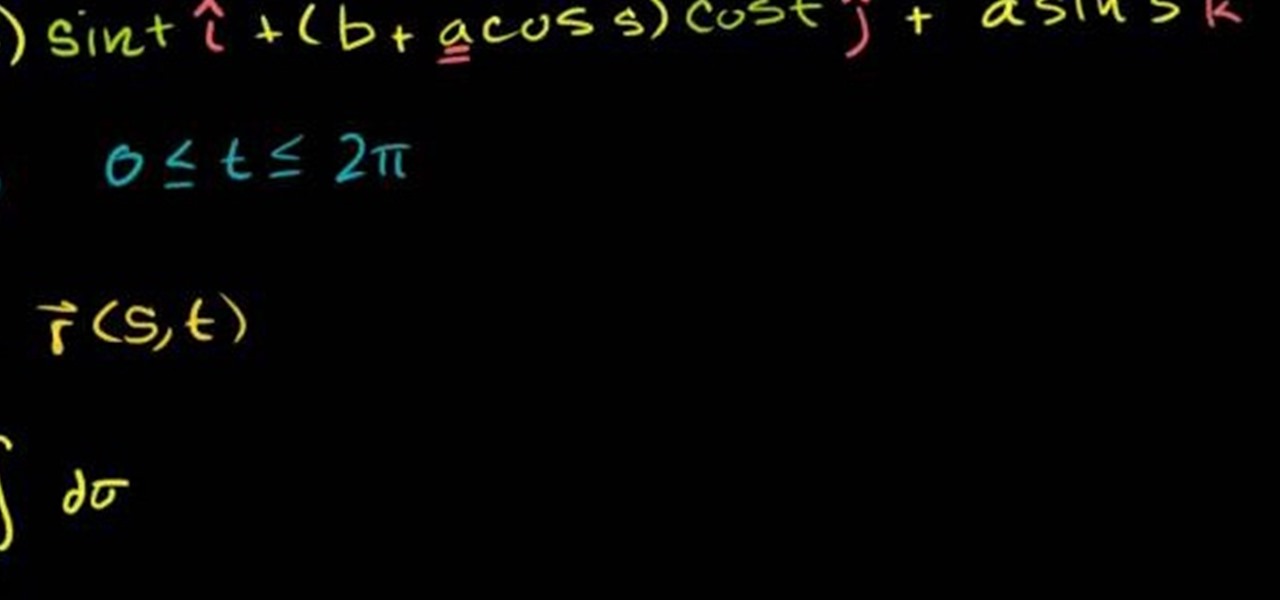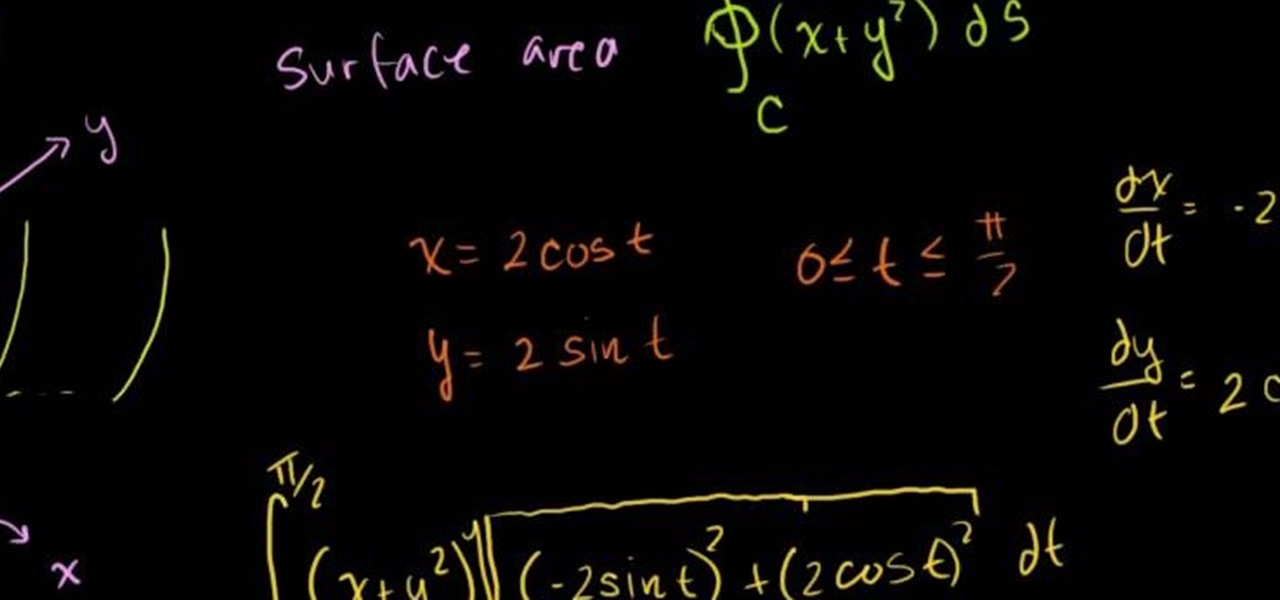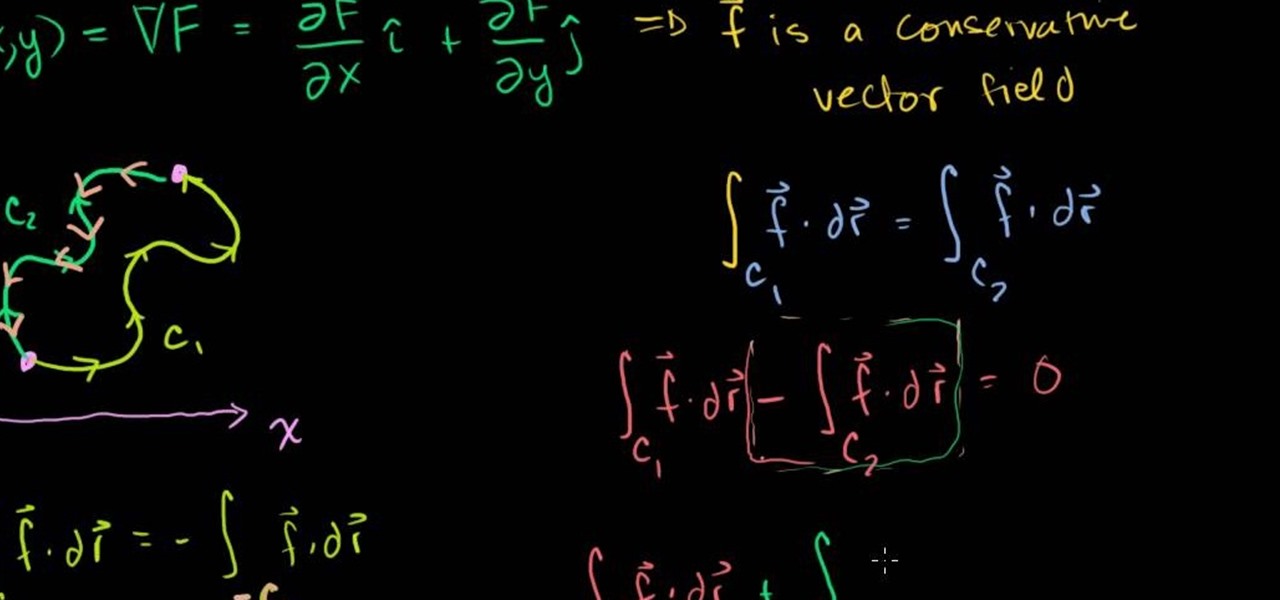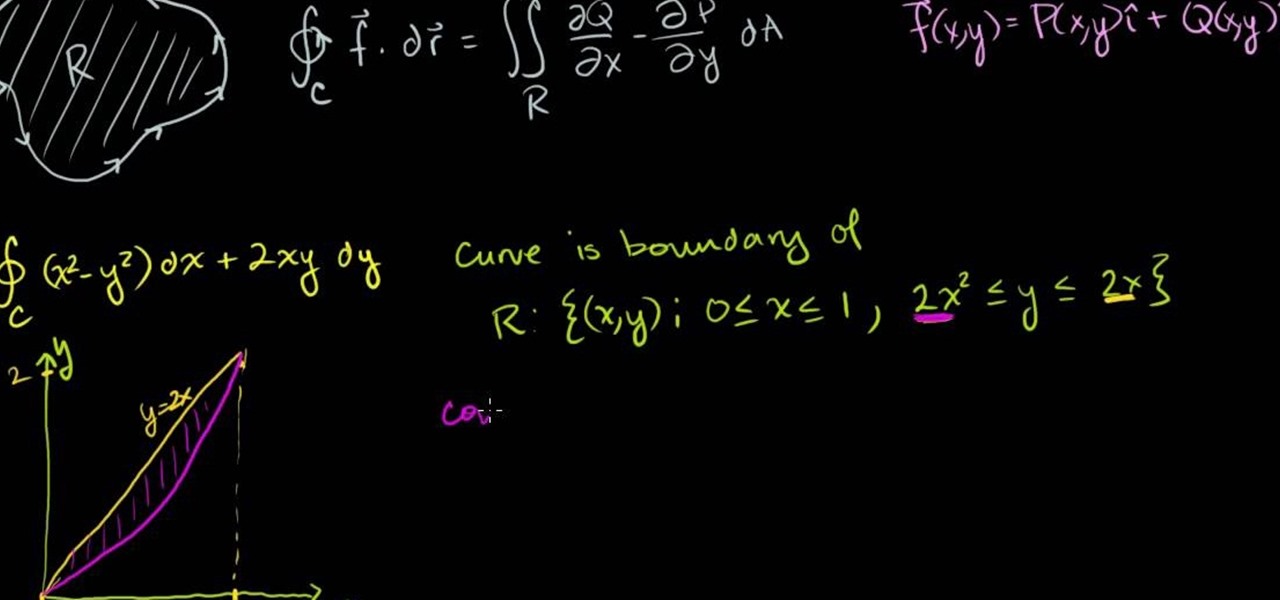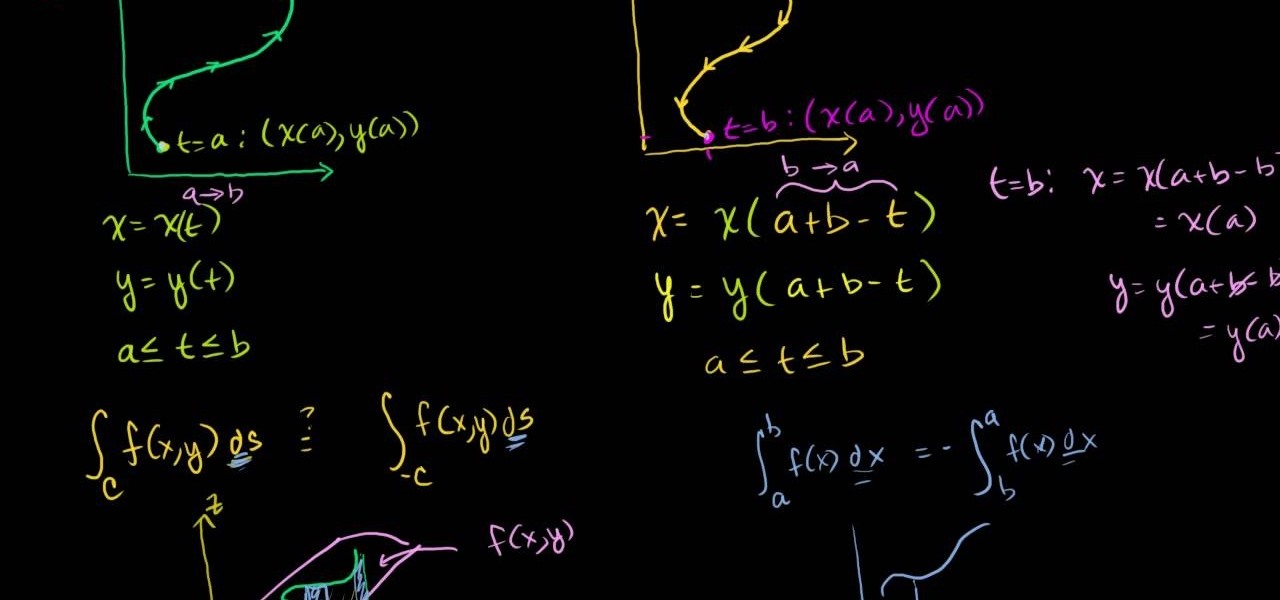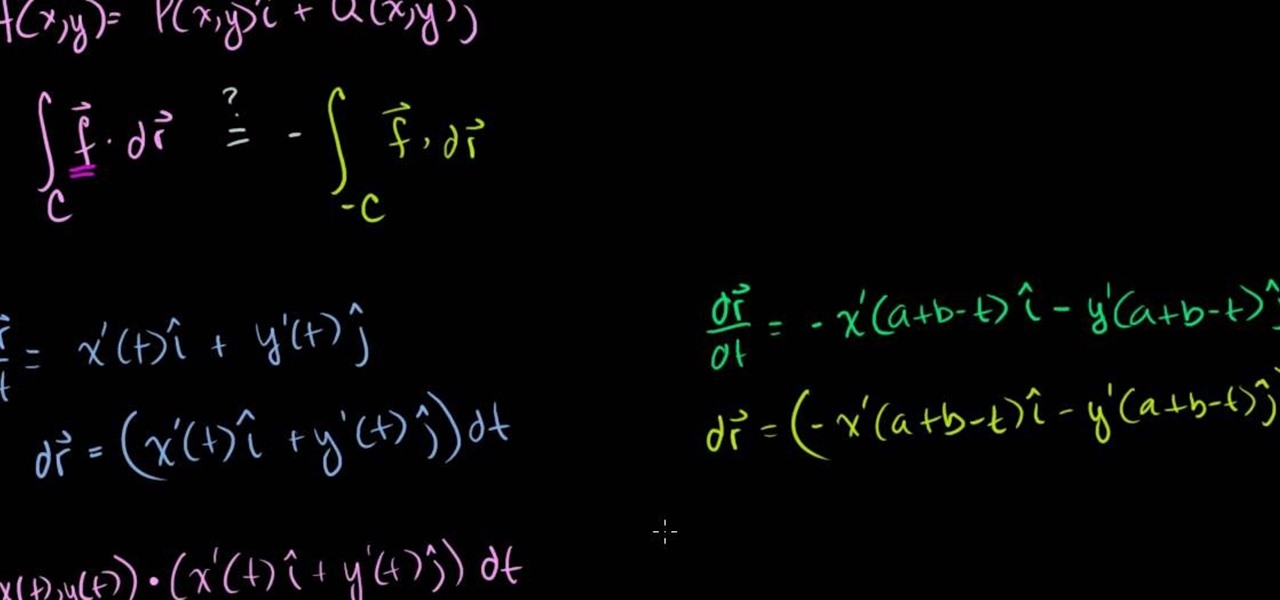
This video demonstrates that, unlike line integrals of scalar fields, line integrals over vector fields are path direction dependent. See how to solve vector-field integrals with this free video calculus lesson. From Ramanujan to calculus co-creator Gottfried Leibniz, many of the world's best and brightest mathematical minds have belonged to autodidacts. And, thanks to the Internet, it's easier than ever to follow in their footsteps (or just finish your homework or study for that next big test).
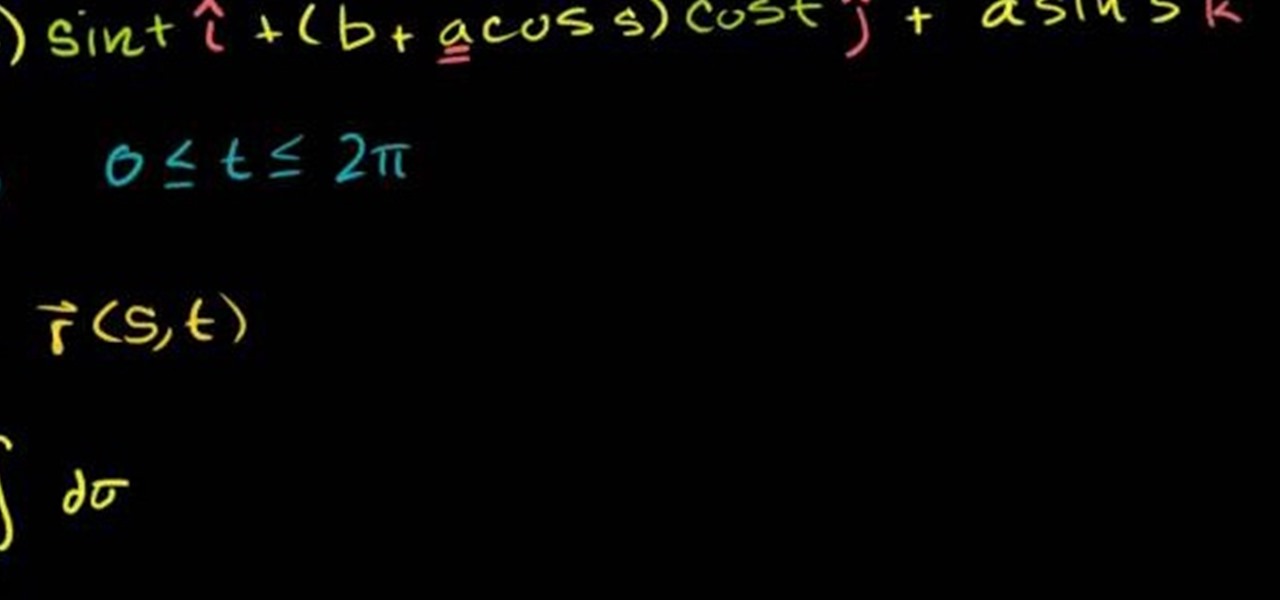
Need help figuring out how to calculate surface integrals in calculus? Learn how. From Ramanujan to calculus co-creator Gottfried Leibniz, many of the world's best and brightest mathematical minds have belonged to autodidacts. And, thanks to the Internet, it's easier than ever to follow in their footsteps (or just finish your homework or study for that next big test). With this installment from Internet pedagogical superstar Salman Khan's series of free math tutorials, you'll learn how to fin...
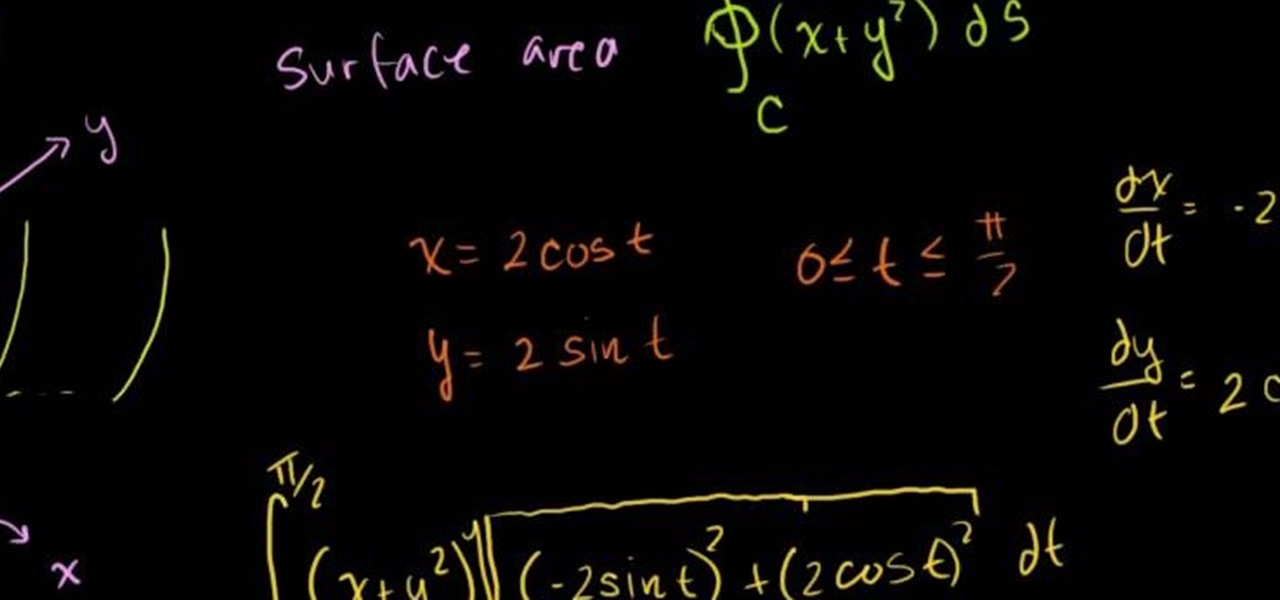
Line integrals will no longer be the feared terrorist of the math world thanks to this helpful guide from the Khan Academy. Marvel at the ease in which the integral is taken over a closed path and solved definitively. Then take out a sheet of paper and see if you can do the same.

Brightstorm explains how to use definite integrals to find area. There are two basic area problems: one in which the area lies between the function and the x-axis, and one where the area lies between the two functions. In the first case, if the curve lies above the x-axis for x=a to x=b, the definite integral returns the area. If it lies below the x-axis for x=a to x=b, then the definite integral returns a negative area. In the second case, we assume that f(x) is the higher curve and g(x) is ...

In this video the math teacher explains the meaning of the indefinite integral formula in calculus by stating it in terms of antiderivatives. He then shows in general terms how this indefinite integral formula has a related derivative formula, i.e. if the derivative of F(x) is f(x) then the indefinite integral of f(x) is F(x) plus a constant term (C). With this general relationship the teacher takes us through three related examples where he converts a derivative formula to an integral formul...
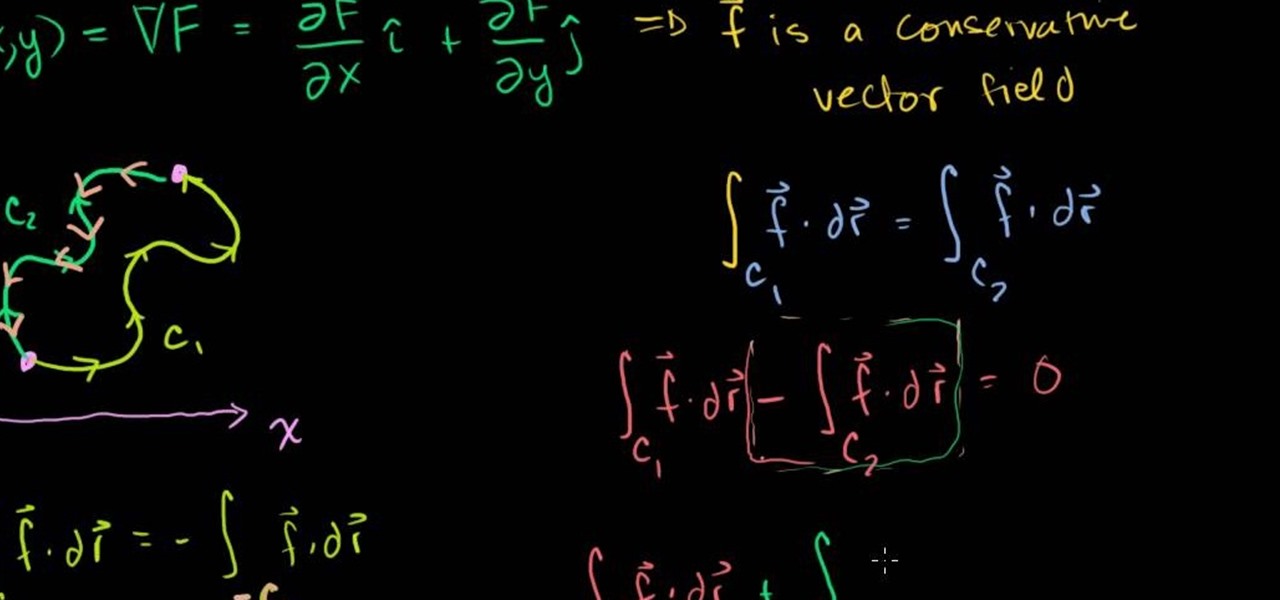
This three part video walks you through the steps of solving closed line integrals on conservative fields. This excellent video shows you a clean blackboard, with the instructors voice showing exactly what to do. Don't fret, any question you may have, will be answered. Watching this video will make you feel like your back in the classroom but rather comfortably from your home.

This three part video walks you through using Green's theorem to solve a line integral. This excellent video shows you a clean blackboard, with the instructors voice showing exactly what to do. Don't fret, any question you may have, will be answered. Watching this video will make you feel like your back in the classroom but rather comfortably from your home.

This video tutorial is in the Education category which will show you how to evaluate a line integral along a straight line segment using the definition of the line integral. This video evaluates a line integral along a straight line segment using a parametric representation of the curve (using a vector representation of the line segment) and then integrating. A vector representation of a line that starts at r0 and ends at r1 is r(t) = (1-t)r0 + tr1 where t is greater than equal to 0 and lesse...

This video demonstrates that the line integral of a scalar field is independent of path direction. Learn how to solve scalar-field integrals with this free video calculus lesson. Looking for a primer on how to solve matrix problems using a TI-89 graphing calculator? See how it's done with this free video algebra lesson. From Ramanujan to calculus co-creator Gottfried Leibniz, many of the world's best and brightest mathematical minds have belonged to autodidacts. And, thanks to the Internet, i...

In this video tutorial, viewers learn how to define an indefinite integral in calculus. Finding the anti-derivatives of a function is called anti-differentiation. The f(x) is the derivative of F(x), while F(x) is the anti-derivative of f(x). If F(x) is the anti-derivative of f(x), then F(x) + c are all anti-derivatives of f(x). This video is very descriptive and clearly explains all the functions of this mathematical function. This video will benefit those viewers who are students, and are in...

See how to approximate a definite integral to a desired accuracy using Maclaurin/Taylor series and the alternating series estimation theorem with this free video calculus lesson. See how it's done with this free video algebra lesson. From Ramanujan to calculus co-creator Gottfried Leibniz, many of the world's best and brightest mathematical minds have belonged to autodidacts. And, thanks to the Internet, it's easier than ever to follow in their footsteps (or just finish your homework or study...

Need to calculate the area between two curves? This free video lesson will show you how. From Ramanujan to calculus co-creator Gottfried Leibniz, many of the world's best and brightest mathematical minds have belonged to autodidacts. And, thanks to the Internet, it's easier than ever to follow in their footsteps (or just finish your homework or study for that next big test). With this installment from Internet pedagogical superstar Salman Khan's series of free math tutorials, you'll learn how...

Want to know how to find the indefinite integral (or anti-derivative) of a polynomial? From Ramanujan to calculus co-creator Gottfried Leibniz, many of the world's best and brightest mathematical minds have belonged to autodidacts. And, thanks to the Internet, it's easier than ever to follow in their footsteps (or just finish your homework or study for that next big test). With this installment from Internet pedagogical superstar Salman Khan's series of free math tutorials, you'll see how to ...

Need help figuring out how to calculate indefinite integrals or antiderivatives in calculus? From Ramanujan to calculus co-creator Gottfried Leibniz, many of the world's best and brightest mathematical minds have belonged to autodidacts. And, thanks to the Internet, it's easier than ever to follow in their footsteps (or just finish your homework or study for that next big test). With this installment from Internet pedagogical superstar Salman Khan's series of free math tutorials, you'll learn...

The lasers from Halo might not be quite as famous as the assault rifles form the games, but they are still a very cool-looking and integral part of it. This video will show you how to make your own replica of the Spartan laser from Halo out of a toy gun called the Belt Blaster. The end result looks so real it might get you arrested by any cops from the future that you may run into, so be careful.

This video demonstrates how to do anti-differentiate functions with radicals in calculus. To simply problems, try to substitute. For example, in the problem, the integral of x times the square root of x plus 2 dx. You can substitute w for everything underneath the radical: i.e. x + 2. When you simplify, it becomes: the integral of x times the square root of w dw. Now, you need to get rid of the x, which you can do with a substitution: w – 2 = x. Now, plug in the values and simplify it.

The reverse toe bounce is a pretty easy freestyle soccer move in which the player juggles the ball up, cross a leg over the other, lets the ball toe bounce on their inside toe, then uncrosses. Using you toe to keep the ball of the ground while your leg spins is an integral element of freestyle soccer, best get good at it.

In this piano music theory lesson you'll go over something of a more advanced piece of music theory: compound intervals.

Square lashing is a knot-making technique that has been made famous by the Boy Scouts of America's massive pioneering projects, and is really integral to any structure made out of poles like the one you see in the thumbnail. This video will teach you how to use square lashing to bind two poles together perpendicular to one another. You will need to know how to tie a clove hitch to make this work.

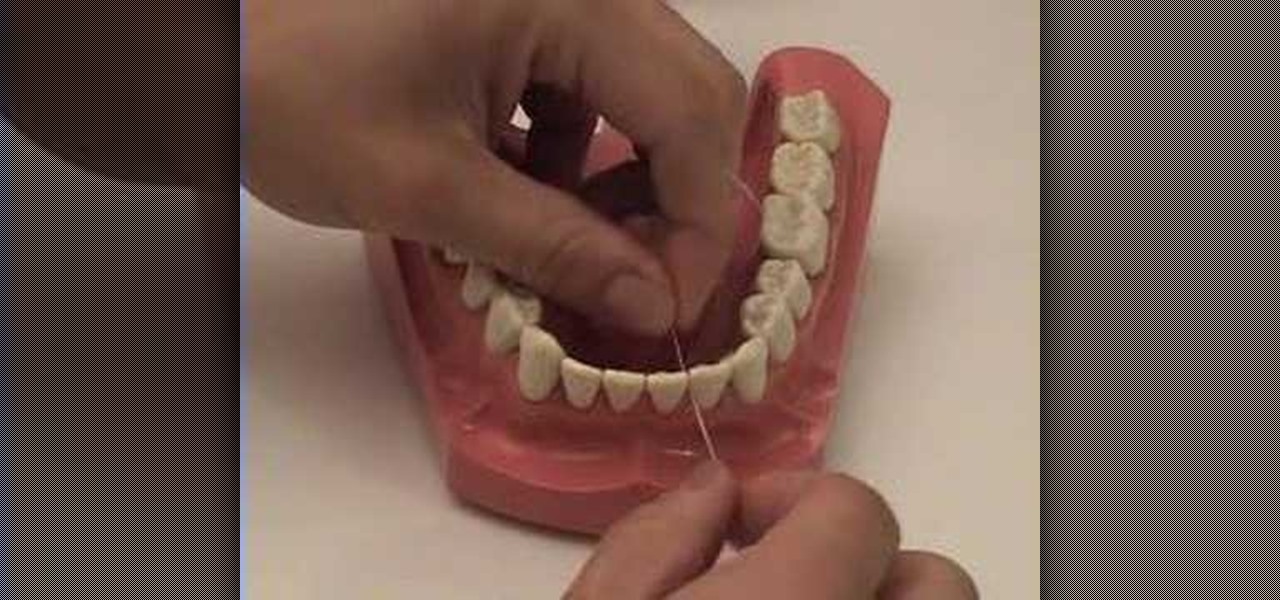
This dental health flossing tutorial illustrates the C-Shape flossing technique for healthy mouth, gums and teeth. Flossing is integral to dental health and keeping your smile beautiful. The demo is done by flossing a pair of false teeth. Floss everyday!

With the noble pursuit of science in mind, Johnny Knoxville learns how to artificially inseminate a cow firsthand. This is an integral part of cattle raising and dairy farming using bull sperm.

This step by step video will show you how to reassemble an Xbox 360 Controller after completing a custom paint job or mod. This is integral and the final step of any great mod, paint or customization job.

There are different techniques to apply the glue on the latex sheeting, none of them is right or wrong. All that matters is what works best for you. This latex tip is helpful for making fetish clothing or latex fashion pieces. Latex clothing is integral to fetish clothing and used frequently in BDSM or S&M sex.

Check out this martial arts tutorial video that demonstrates how to throw your opponent. Throws are an integral part of jujitsu and all martial arts. Get expert tips and advice on grappling in this instructional Jiu-Jitsu video. There are a lot of ways to get your opponent off balance. Learn how with this video and practice your Jiu-Jitsu sills and techniques.

Mary Janes are the classic American girl shoe, their style simplistic yet subtly feminine, polished and clean. Perfect for parties but also with punk rock potential when dressed down, these shoes are integral pieces in a girl's wardrobe.

This is a tutorial of the No Handed Kick (Kip) Up Trick. It is integral to breakdancing, capoeira, parkour and gymnastics. Get to your feet without hands. Some people do hand kip ups that have them roll on their neck and push up overhanded. That's lame. This no-handed version is rad.

This is a collected tutorial video from the "Ottawa's Eddy Matzger Weekend" event. Featuring Barry Publow and Eddy Matzger, learn how to properly crossover in competitive speed inline skating. The crossover is integral to getting fluidity in the motion and to make smooth turns or gain speed.

Here is a video of basic glowchuck movements. This is a liquid dance prop dance instructional. Using glowsticks in liquid dance is integral especially for electronic parties and rave nights. It adds a textural element to dancing in crowds.

This is an instructional video featuring Doug Prime, founder of the Future Engineers Center at the University of Massachusetts Lowell. This is an instructional on utility knives that are used to cut foam board and cardboard. The blade replacement is integral to this craft or hobby tool's maintenance.

Watch this video to learn how to use class in C++. Classes are an integral part of object oriented programming.

Lemon Rice is one of the many rice dishes that are an integral part of South Indian Cuisine. It is a flavorful tangy twist to plain white rice.

Paratha are an Indian unleavened bread and an integral part of many Indian meals. Any subzi (vegetable) or curry dish can be fabulous when accompanied with fresh, hot parathas. Note: Chapatis are cooked without oil whereas Parathas are pan fried.

Having a backup sump pump is integral in making sure your basement is always protected from water, and if you have the Basement Watchdog battery backup sump pump system, this video will show you the complete installation procedure. Find out how to build a pipe connection to the discharge pipe, install check valves, connect to the backup system and more.

Though purchasing pretty eyeshadows and makeup palettes at Sephora is much more exciting, selecting the right makeup brushes and learning how to use each one when applying the sparkly goodness from these eyeshadow tins and palettes is important to achieve a cohesive, non-clownish look.

This tutorial video will show you how to do one of the most basic elements of cup stacking: the three cup stack. The three cup stack is an integral part of advanced cup stacking.

When you have to find the average of two or five numbers then you have to add all the numbers and then divide then by two or five respectively. However in the case of the function you have infinite many values over any interval. But it is not possible to add all and then divide by infinity. Therefore you have to calculate in a different way. Imagine that you have to find the average of y=f(x). Then you have to average it from the interval from ‘a’ to ‘b’. The inner area under the curve shall ...

Bobby pins have been integral tools in a girl's makeup case since the 1920s, when bobby pins were used to secore the fashionable hair bobs of the Jazz Age. They've also recently been used by young boys and hackers to pick open locks. And now you can add one more fun use to the list.

Snake cams have become the epitome of high-tech gadgets on television and in movies. In 24, Jack Bauer is always using a snake camera to check for terrorists on the other side of the door. The video game Splinter Cell has made the snake cam an integral part to Sam Fisher's arsenal. And almost every spy movie features its use at one point or another.

Ghee is also known as clarified butter and is an integral part of cooking and a must have in most Indian kitchens. It is made from fresh butter that is melted and simmered to get a clear golden liquid. You can use the ghee to season lentils, vegetables, to make parathas, sweets or even use it as a spread.

This video is about deriving the area of a circle of radius 'r' using polar co-ordinate. First, we draw a circle and its radius 'r'. Then draw another radius close to it, so that it forms a small triangle-like figure. To find the area of the complete circle, divide the circle into similar small triangles. The area of each triangle is given by half the product of its perpendicular and the base. We give the angle between the two radii as d?. We get the area of the small triangle by substituting...